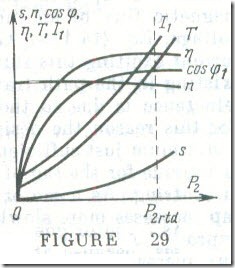
The speed-torque characteristic of an induction motor is a convenient indicator of its properties as a part of an electric drive. A deeper insight into its performance, however, can be gleaned from what we call its operating characteristics which relate its speed n , its torque T, its power factor cos φ and its efficiency η = P2/P1 to its output power (that is, the power available at its output shaft), P2 . The operating characteristics are plotted at the rated supply frequency f and at the rated stator terminal voltage V1 = V1,rtd .
Since P2 ≈ Pm , and Pm = Tɷrot , It follows that the speed characteristic n = f (P2) differs but little from the speed-torque characteristic of the same motor, and it may likewise be called flat (Fig 29) .
The torque T developed by an induction motor is the sum of the useful torque T2 (utilized to overcome the load applied to its output shaft) and the no-load torque Tnl (which goes to make up for the mechanical losses in the motor). The no-load torque may be assumed to be independent of the load sustained by the motor. The useful torque is given by
T2 = P2/ɷrot
and if ɷrot were always the same, the relation T2 = f (P2) would be linear. Actually, however, the angular velocity of the motor slightly falls off with increasing P2 so the plot of T2 as a function of P2 bends upwards as slightly. In consequence, the plot of T as a function of P2 , which is a combination of the plots of Tnl and T2 as functions of P2, intersects the axis of ordinates at a point corresponding to Tnl past which it remains nearly flat and only slightly bends upwards.
As the load on an induction motor changes, the power factor cos φl varies due to the following causes. The magnetizing current of an induction motor depends slightly on load, because an increase in it only leads to an increase in the leakage flux linkages which are proportional 1.0 the currents in the stator and rotor windings, whereas the main magnetic flux of the machine goes down a little as the load is increased. On the other hand, the current in the motor is proportional to its mechanical load. Thus, as the load on a motor is increased, the relative value of reactive current falls off at a high rate but the power factor cos φ1 increases. At no load, the power factor of an induction motor is low, being about 0.2. As the load goes up, the power factor increases, too, and achieves its maximum value (0.7-0.9) at a load close to the rated one. As a result, the reactive current accounts for anywhere between 40 and 70% of the stator current even in the case of a motor running at full load.
The fact that induction motors are never loaded to their full capacity is one of the principal causes why industrial networks operate at a low power factor. A natural way to improve the power factor would be to operate induction motors at full rated load. The main magnetic flux of an induction motor is proportional to its stator voltage, Eq. (14.11b). Given a fixed value of flux, the magnetizing current exciting this flux is inversely proportional to the reluctance existing in the path traversed by the flux. The greater part of this reluctance is due to the air gap between the stator and the rotor. For this reason the designer usually strives to keep this air gap to a minimum just sufficient to assure proper operation of the bearings, to provide for the sag of the shaft, and to accommodate likely errors in centring . As a motor goes up in power rating, the necessary air gap increases more slowly than the power rating, so its power factor improves. As a motor goes down in rated speed, the main magnetic flux increases, because at a lower speed it induces a lower emf in the stator phases. As a consequence, low-speed motors have a relatively heavier magnetizing current and a substantially lower power factor.
The efficiency of an induction motor is defined as the ratio of the output power (that which is available at its shaft) P2 to the power Pl it draws from the supply line
η = P2/P1
The input power Pl is the sum of the useful or output power P2 and of all the losses
P1 = P2 + Ploes
The losses occurring in a motor may be divided into the fixed (or constant) losses which are independent of load, and the variable loss which is dependent on load.
The fixed (or constant) losses combine the power lost in the stator core due to hysteresis and eddy currents, and mechanical losses found from a no-load test .
The variable loss occurring in a motor is due to the power dissipated as heat in the stator and rotor windings
Pv1 = 3rW1I21
and
PV2 = m2rw2I22
The efficiency of an induction motor is a maximum (65-95 %) when the variable loss is equal to the fixed losses (see Sec. 8.9). For most motors, the maximum efficiency occurs at a load equal to 75 % of the rated one, because motors are designed in view of the fact that they are not always operated at full load.
Labels: Electricity and Magnetism