In This Chapter:
✔ Centripetal Acceleration
✔ Centripetal Force
✔ Motion in a Vertical Circle
✔ Gravitation
✔ Satellite Motion
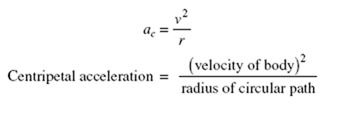
Centripetal Acceleration
A body that moves in a circular path with a velocity whose magnitude is constant is said to undergo uniform circular motion.
Although the velocity of a body in uniform circular motion is constant in magnitude, its direction changes continually. The body is there- fore accelerated. The direction of this centripetal acceleration is toward the center of the circle in which the body moves, and its magnitude is
Note!
Centripetal Force
The inward force that must be applied to keep a body moving in a circle is called centripetal force. Without centripetal force, circular motion can- not occur. Since F = ma, the magnitude of the centripetal force on a body in uniform circular motion is

(b) The centripetal force on a car making a turn on a level road is provided by the road acting via friction on the car’s tires.
Motion in a Vertical Circle
When a body moves in a vertical circle at the end of a string, the tension T in the string varies with the body’s position. The centripetal force Fc on the body at any point is the vector sum of T and the component of the body’s weight w toward the center of the circle. At the top of the circle, as in Figure 6-1(a), the weight w and the tension T both act toward the center of the circle, and so
T = Fc − w
At the bottom of the circle, as in Figure 6-1(b), w acts away from the center of the circle, and so
T = Fc + w
Figure 6-1
Solved Problem 6.2 A string 0.5 m long is used to whirl a 1-kg stone in a vertical circle at a uniform velocity of 5 m/s. What is the tension of the string (a) when the stone is at the top of the circle and (b) when the stone is at the bottom of the circle?
Solution.
(a) The centripetal force needed to keep the stone moving at 5 m/s is
The weight of the stone is w = mg = (1 kg)(9.8 m/s2) = 9.8 N. At the top of the circle,
T = Fc − w = 50 N − 9.8 N = 40.2 N
(b) t the bottom of the circle,
T = Fc + w = 59.8 N
According to Newton’s law of universal gravitation, every body in the universe attracts every other body with a force that is directly proportional to each of their masses and inversely proportional to the square of the distance between them. In equation form,
where m1 and m2 are the masses of any two bodies, r is the distance be- tween them, and G is a constant whose values in SI and British units are, respectively,
SI units: G = 6.67 × 10−11 N · m2/kg2
British units: G = 3.34 × 10−8 lb · ft2/slug2
A spherical body behaves gravitationally as though its entire mass were concentrated at its center.
Solved Problem 6.3 What gravitational force does a 1000-kg lead sphere exert on an identical sphere 3 m away?
Solution.
This is less than the force that would result from blowing gently on one of the spheres. Gravitational forces are usually significant only when at least one of the bodies has a very large mass.
Gravitation provides the centripetal forces that keep the planets in their orbits around the sun and the moon in its orbit around the earth. The same is true for artificial satellites put into orbit around the earth.
Labels: Classical mechanics