Much of the electrical energy used worldwide is produced by alternating-current (ac) generators. Such widespread use of alternating current means that students in electrical trades must understand the principles of electricity and magnetism and their application to alternating-current circuits, components, instruments, transformers, alternators, ac motors, and control equipment.
Uses for Direct Current
Although alternating current is more commonly used, there are a number of applications where direct current (dc) either must be used or will do the job better than alternating current. Several of these applications are described in the following list:
• Direct current is used for various electrochemical processes, including electroplating, refining of copper and aluminum, electrotyping, production of industrial gases by electrolysis, and charging of storage batteries.
• Direct current is used to excite the field windings of alternating-current generators.
• Direct current applied to variable speed motors results in stepless, precise speed adjustments. Such motors are used in metal rolling mills, papermaking machines, high-speed gearless elevators, automated machine tools, and high-speed printing presses.
• Traction motors require direct current. Such motors are used on locomotives, subway cars, trolley buses, and large construction machinery that will not be driven on high- ways. Using a dc motor in these applications eliminates the need for clutches, gear shifting transmissions, drive shafts, universal joints, and differential gearing. Thus, almost all large locomotives have diesel engines that drive direct-current generators to supply the power for dc traction motors installed in each locomotive truck.
Under normal conditions, the electrical energy produced by alternating-current generators is transmitted to the areas where it is to be used by alternating-current loads. If direct current is required, the alternating current is changed to direct current by rectifiers or motor–generator sets. Fortunately, alternating current is suited for use with heating equipment, lighting loads, and constant-speed motors. Loads of this type are the most common users of electrical energy. Thus, the costly conversion to direct current is needed only for certain load requirements.
Advantages of Alternating Current
Alternating current is preferred to direct current for large generating, transmission, and distribution systems for the following reasons:
• AC generators can be built with much larger power and voltage ratings than dc genera- tors. An ac generator does not require a commutator. The armature or output winding of the ac generator can be mounted on the stator (stationary part) of the machine. The output connections are made with cables or bus bars bolted directly to the stator windings (stationary armature windings). Armature voltages of 13,800 volts or more are common. Currents of any desired value can be obtained with the proper machine design. The rotating member of the alternator is the field. This field is supplied with direct current by means of slip rings or by means of a brushless exciter from an external dc source. The voltage of the source is in the range of 100 to 250 V. In contrast to the ac generator, the armature or output winding of a dc generator must be the rotating part of the machine. The connection of the armature to the external load is made through a commutator and brushes. These components restrict the maximum voltages and cur- rents that can be obtained from dc machines to practical levels. Large dc machines rated at 600 to 750 V are common. Occasionally, a machine rated at 1500 V is required
for certain applications. The commutators of dc generators are usually rated at less than 8000 amperes (A). These large current ratings are practical only on slow-speed machines. For these reasons, dc generator ratings are limited to relatively low voltage and power values as compared to ac generators.
• With the use of alternating current, the voltage can be stepped up or stepped downefficiently by means of transformers. A transformer has no moving parts, and its losses are relatively low. The efficiency of most transformers at the rated load is high, from 95% to more than 99%. Transformers cannot be used with direct current. DC voltage changes are obtained by using series resistors, which give rise to I2R losses, or motor– generator sets, which have relatively low overall efficiencies. However, the reduction or increase of dc voltages in dc systems is inefficient.
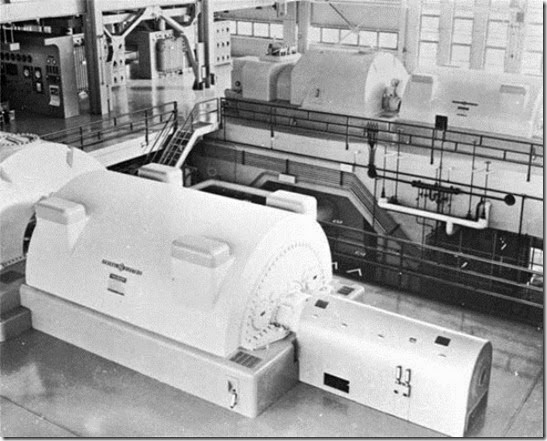
• Large ac generators having very high power ratings (Figure 1–1), plus efficient trans- formers to step up or step down the alternating voltage, make it possible to conduct
FIGURE 1–1 A 44,000 kW power company installation (Courtesy of General Electric Company)
ac energy economically over long distances from generating stations to the various load centers by way of high-voltage transmission systems. Thus, huge amounts of electrical energy can be generated at one location. For example, a large hydroelectric generating station may be located near a waterfall. Here, the energy can be generated at a relatively low cost per kilowatt-hour. Large steam-generating stations are also located where fuel is easy to obtain and abundant water is available. Steam-generating stations use very large-capacity alternators having efficiency ratings as high as 97%. Large high-speed turbines operating at very high steam pressures are used to turn the ac generators. The efficiency of these steam turbines, operating at speeds of 1200, 1800, or 3600 revolutions per minute (r/min), is much greater than that of steam turbines used in smaller generating plants. Completely automated control systems are used in modern generating stations to increase the total operating efficiency even more. As a result, large steam-generating plants and hydroelectric stations operate efficiently to produce electrical energy at a low generating cost per kilowatt-hour.
• The ac induction motor (Figure 1–2) has no commutator or brushes. This type of motor has a relatively constant speed. It is rugged and simple in construction. The initial purchase price and the maintenance and repair costs for the ac induction motor are considerably less than the costs for a dc motor of comparable horsepower, voltage, and speed. Further, the starting equipment used with a typical induction motor is also lower in cost initially when compared to the starting equipment used with dc motors having similar horsepower ratings. Because ac induction motors do not contain a commutator or brushes, they generally have a longer life span and require less maintenance than dc machines.
FIGURE 1–2 Cutaway view of a squirrel cage induction motor (Courtesy of General Electric Company)
A basic knowledge of trigonometry is essential to an understanding of alternating-current concepts. That is, the student must know the basic mathematical relationships between right triangles and angles in the quadrants of a coordinate system.
Coordinate System and Angular Relationships
Figure 1–3 shows a coordinate system consisting of an X axis and a Y axis. These axes are mutually perpendicular lines that form four 90° angles called quadrants. Quad- rants 1 through 4 in the figure are numbered counterclockwise.
Figure 1–4 represents a given X–Y coordinate system. The indicated angles are measured from the positive X axis to a given line. Lines OA and OB (quadrant 1) (Figure 1–4A) form a 90° angle at 0. Line OC (quadrant 1) and line OD (quadrant 2) (Figure 1–4B) form a 120° angle at 0. In Figure 1–4C, lines OE (quadrant 1) and OF (quadrant 3) form a 240°
angle at 0. In these examples, all of the angles are measured from the positive (+) X axis to the indicated line in the counterclockwise direction. If the angle is measured in the clockwise direction from the positive X axis, the angle is negative because the direction of measurement has changed. (See line OF in Figure 1–4C.) A simple saying can be used to help remember the relationships of sine, cosine, and tangent. Use the first letter of each word in the saying, “Oscar Had A Heap Of Apples” in Figure 1-5B.
Figures 1–5A and 1–6 summarize the angular relationships for the various quadrants. These relationships will be used throughout this text in vector problems.
Generation of Sine and Cosine Waves
Figures 1–3 and 1–4 showed the static positions of a line in different quadrants. If the line is allowed to rotate counterclockwise, an analysis can be made of the projections (or shadows) of the line on the X and Y axes (Figure 1–7). A wave called a sine wave and one called a cosine wave will be generated by the rotating line.
The student should be able to visualize the shadow of the line as it rotates. As the angle theta (8) increases, the shadow of the line on the Y axis increases and the shadow of the line on the X axis decreases.
Figure 1–8 shows the pattern obtained by rotating a line of magnitude R about the 0 point. The projections of R on the Y axis are plotted against the angle theta (8) made by the line as it moves from the positive X axis. The wave pattern formed is called a sine wave and is expressed by the formula y = R sin 8, where R is the radius of the circle, 8 is the angle moved (traversed) by the line from the positive X axis, and Y is the projection or shadow of the line on the Y axis.
In a similar manner, the projection of the rotating line on the X axis can be plotted against the angle 8 made by the line as it rotates. Figure 1–9 shows the resulting wave pattern.
In Figure 1–9, the projection of the radius (R) on the X axis is zero at angles of 90° and 270°. The resulting wave pattern is called a cosine wave. This waveform is expressed by the formula y = R cos 8, where R is the radius of the circle.
Comparing the two wave patterns, it can be seen that when one has a magnitude of zero, the other has the maximum magnitude R, and vice versa. Note that the cosine wave has the same pattern as the sine wave, but reaches its maximum value 90° before the sine wave.
Sine and cosine waves can be generated at the same time by rotating two lines that are 90° out of step or out of phase with each other. The projections of these two lines on the Y axis can be plotted against the common angle of movement (8). Figure 1–10 shows the waveforms generated in this manner. The intermediate points have been deleted to reduce confusion in the drawing.
There may be some confusion because of the two angles marked 8 in Figure 1–10.
Recall that all angles are measured from the positive X axis. In this case, the angle 8 rep- resents not only the movement of line A to A' but also the movement of the A–B structure from the A–B position to the A'–B' position. The structure has moved an angle 8, which is measured from the positive X axis. Line OB generates a cosine wave, and line OA generates a sine wave.
A discussion follows of how alternating voltages are generated using the principles learned in the study of direct current. This discussion will show that the sine wave is not generated by means of projections of a moving line on the Y axis. Rather, the sine wave is a function of the position of a coil in a magnetic field. One important point should be kept in mind: the mathematical relationships of all sine waves are the same, regardless of the method by which they are generated. All sine waves have the same form as expressed by the equation Y = R sin 8.
Labels: Direct current fundamentals