When a conductor is moved across a magnetic field so as to cut through the lines of force (or flux), an electromotive force (e.m.f.) is produced in the conductor. If the conductor forms part of a closed circuit then the e.m.f. produced causes an electric current to flow round the circuit. Hence, an e.m.f. (and thus current) is ‘induced’ in the conductor as a result of its movement across the magnetic field. This effect is known as ‘electromagnetic induction’.
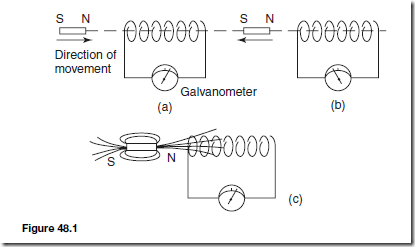
Figure 48.1(a) shows a coil of wire connected to a centre-zero galvanometer, which is a sensitive ammeter with the zero-current position in the centre of the scale.
(a) When the magnet is moved at constant speed towards the coil (Figure 48.1(a)), a deflection is noted on the galvanometer showing that a current has been produced in the coil.
(b) When the magnet is moved at the same speed as in (a) but away from the coil the same deflection is noted but is in the opposite direction (see Figure 48.1(b)).
(c) When the magnet is held stationary, even within the coil, no deflection is recorded.
(d) When the coil is moved at the same speed as in (a) and the magnet held stationary the same galvanometer deflection is noted.
(e) When the relative speed is, say, doubled, the galvanometer deflection is doubled.
(f) When a stronger magnet is used, a greater galvanometer deflection is noted.
(g) When the number of turns of wire of the coil is increased, a greater galvanometer deflection is noted.
Figure 48.1(c) shows the magnetic field associated with the magnet. As the magnet is moved towards the coil, the magnetic flux of the magnet moves across, or cuts, the coil. It is the relative movement of the magnetic flux and the coil that causes an e.m.f. and thus current, to be induced in the coil. This effect is known as electromagnetic induction. The laws of electromagnetic induction evolved from experiments such as those described above.
Faraday’s laws of electromagnetic induction state:
(i) An induced e.m.f. is set up whenever the magnetic field linking that circuit changes.
(ii) The magnitude of the induced e.m.f. in any circuit is proportional to the rate of change of the magnetic flux linking the circuit.
Lenz’s law states:
The direction of an induced e.m.f. is always such that it tends to set up a current opposing the motion or the change of flux responsible for inducing that e.m.f.
An alternative method to Lenz’s law of determining relative directions is given by Fleming’s Right-hand rule (often called the gene Rator rule) which states:
Let the thumb, first finger and second finger of the right hand be extended such that they are all at right angles to each other (as shown in Figure 48.2). If the first finger points in the direction of the magnetic field and the thumb points in the direction of motion of the conductor relative to the magnetic field, then the second finger will point in the direction of the induced e.m.f.
Summarising:
First finger — Field
ThuMb— Motion
SEcond finger — E.m.f.
In a generator, conductors forming an electric circuit are made to move through a magnetic field. By Faraday’s law, an e.m.f. is induced in the conductors, and thus a source of e.m.f. is created. A generator converts mechanical energy into electrical energy. (The action of a simple a.c. generator is described in Chapter 54).
The induced e.m.f. E set up between the ends of the conductor shown in Figure 48.3 is given by:
where B, the flux density, is measured in teslas, l, the length of conductor in the magnetic field, is measured in metres, and v, the conductor velocity, is measured in metres per second.
If the conductor moves at an angle e° to the magnetic field (instead of at 90° as assumed above) then:
For example, a conductor moves with a velocity of 15 m/s at an angle of 90° to a magnetic field produced between two square-faced poles of side length 2 cm. If the flux leaving a pole face is 5 µWb, the magnitude of the induced e.m.f., is given by:
If the conductor moves at an angle of, say, 30° then:
E30 D Blv sin 30° = E90 sin 30° = 3.75 sin 30° = 1.875 mV
Inductance is the name given to the property of a circuit whereby there is an e.m.f. induced into the circuit by the change of flux linkages produced by a current change.
When the e.m.f. is induced in the same circuit as that in which the current is changing, the property is called self inductance, L
When the e.m.f. is induced in a circuit by a change of flux due to current changing in an adjacent circuit, the property is called mutual inductance, M (see chapter 49 following).The unit of inductance is the henry, H.
A circuit has an inductance of one henry when an e.m.f. of one volt is induced in it by a current changing at the rate of one ampere per second
Induced e.m.f. in a coil of N turns,
Inductors
A component called an inductor is used when the property of inductance is required in a circuit. The basic form of an inductor is simply a coil of wire.
Factors that affect the inductance of an inductor include:
(i) the number of turns of wire – the more turns the higher the inductance
(ii) the cross-sectional area of the coil of wire — the greater the cross-sectional area the higher the inductance
(iii) the presence of a magnetic core – when the coil is wound on an iron core the same current sets up a more concentrated magnetic field and the inductance is increased
(iv) the way the turns are arranged– a short thick coil of wire has a higher inductance than a long thin one.
Two examples of practical inductors are shown in Figure 48.4, and the standard electrical circuit diagram symbols for air-cored and iron-cored inductors are shown in Figure 48.5.
An iron-cored inductor is often called a choke since, when used in a.c. circuits, it has a choking effect, limiting the current flowing through it.
Inductance is often undesirable in a circuit. To reduce inductance to a minimum the wire may be bent back on itself, as shown in Figure 48.6, so that the magnetising effect of one conductor is neutralised by that of the adjacent conductor. The wire may be coiled around an insulator, as shown,
without increasing the inductance. Standard resistors may be non-inductively wound in this manner.
An inductor possesses an ability to store energy. The energy stored, W, in the magnetic field of an inductor is given by:
For example, the energy is stored in the magnetic field of an 8 H inductor which has a current of 3 A flowing through it, is given by: